Equivalent Fractions

Equivalent Fractions have the same value, even though they may look different.

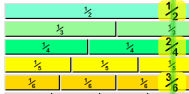
These fractions are really the same:

1/2		2/4		4/8
	=		=

Why are they the same? Because when we multiply or divide both the top and bottom by the same number, the fraction keeps its value, like this:

	× 2		× 2
12   =   24   =   48
	× 2		× 2

The rule to remember is:

"Change the bottom using multiply or divide,
And the same to the top must be applied"

Note: this rule is for multiply or divide, not add or subtract.

See the Chart of Fractions with many examples of equivalent fractions.

And see Fractions on the Number Line ...

... it has a slider

Example: Cooking Measurements

When following a recipe, if we need 12 cup of sugar but only have a 14 cup measuring tool, we can use it twice to achieve the same amount.

This shows that 24 is equivalent to 12

Dividing

Here are some more equivalent fractions, this time by dividing:

	÷ 3		÷ 6
1836   =   612   =   12
	÷ 3		÷ 6

Choose the number to divide by carefully, so that the results (both top and bottom) stay as whole numbers.

If we keep dividing until we can't go any further, then we have simplified the fraction (made it as simple as possible).

Summary:

• You can make equivalent fractions by multiplying or dividing both top and bottom by the same amount.
• You only multiply or divide, never add or subtract, to get an equivalent fraction.
• Only divide when the top and bottom stay as whole numbers.