Theorems about Similar Triangles

1. The Side-Splitter Theorem

triangles similar ABC and ADE

If ADE is any triangle and BC is drawn parallel to DE, then ABBD = ACCE

To show this is true, draw the line BF parallel to AE to complete a parallelogram BCEF:

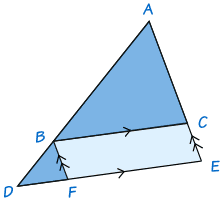
triangles similar ABC and ADE: BF and EC same

Triangles ABC and BDF have exactly the same angles and so are similar (Why? See the section called AA on the page How To Find if Triangles are Similar.)

Side AB corresponds to side BD and side AC corresponds to side BF.
So AB/BD = AC/BF
But BF = CE
So AB/BD = AC/CE

The Angle Bisector Theorem

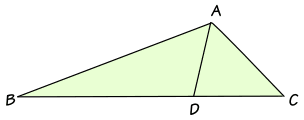
triangles similar ABC point D

If ABC is any triangle and AD bisects (cuts in half) the angle BAC, then ABBD = ACDC

To show this is true, we can label the triangle like this:

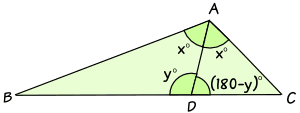
triangles similar angles x and x at A and angles y and 180-y at D

Angle BAD = Angle DAC = x°
Angle ADB = y°
Angle ADC = (180−y)°

By the Law of Sines in triangle ABD:sin(x)BD = sin(y)AB

Multiply both sides by AB:sin(x)AB BD = sin(y)1

Divide both sides by sin(x):ABBD = sin(y)sin(x)

By the Law of Sines in triangle ACD:sin(x)DC = sin(180−y)AC

Multiply both sides by AC:sin(x)ACDC = sin(180−y)1

Divide both sides by sin(x):ACDC = sin(180−y)sin(x)

But sin(180−y) = sin(y):ACDC = sin(y)sin(x)

Both ABBD and ACDC are equal to sin(y)sin(x), so:

ABBD = ACDC

In particular, if triangle ABC is isosceles, then triangles ABD and ACD are congruent triangles

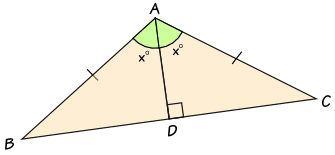
triangles similar right angle at D

And the same result is true:

ABBD = ACDC

3. Area and Similarity

If two similar triangles have sides in the ratio x:y,

then their areas are in the ratio x²:y²

Example:

These two triangles are similar with sides in the ratio 2:1 (the sides of one are twice as long as the other):

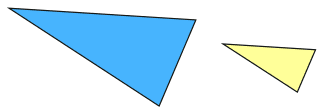
triangles similar large and small

What can we say about their areas?

The answer is simple if we just draw in three more lines:

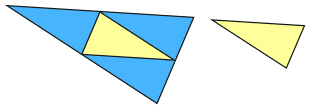
triangles similar small fits inside large 3 times

We can see that the small triangle fits into the big triangle four times.

So when the lengths are twice as long, the area is four times as big

So the ratio of their areas is 4:1

We can also write 4:1 as 2²:1

The General Case:

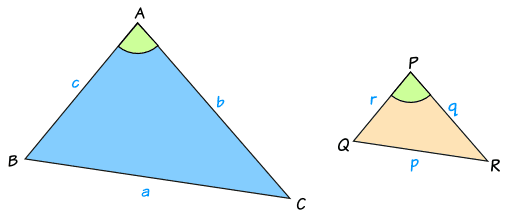
triangles similar ABC and PQR

Triangles ABC and PQR are similar and have sides in the ratio x:y

We can find the areas using this formula from Area of a Triangle:

Area of ABC = 12bc sin(A)

Area of PQR = 12qr sin(P)

And we know the lengths of the triangles are in the ratio x:y

q/b = y/x, so: q = by/x

and r/c = y/x, so r = cy/x

Also, since the triangles are similar, angles A and P are the same:

A = P

We can now do some calculations:

Area of triangle PQR:12qr sin(P)

Put in "q = by/x", "r = cy/x" and "P=A":12(by)(cy) sin(A)(x)(x)

Simplify:12bcy² sin(A)x²

Rearrange:y²x² × 12bc sin(A)

Which is:y²x² × Area of Triangle ABC

So we end up with this ratio:

Area of triangle ABC : Area of triangle PQR = x²: y²

1806, 1807, 3965, 1808, 1809, 3966, 1810, 1811, 3967, 3968